RIFLE BULLETS
Discover All
PISTOL BULLETS
Discover All
RESOURCES
Discover All
DEALER LOCATOR
Discover All
CONFIDENCE IN EVERY SHOT
We test over 250,000 rounds annually in our underground range to ensure every bullet is trusted to perform when it matters most. From hunters to competitive shooters, Sierra offers bullets designed for every pursuit.
Discover MoreSIERRA BOX BULLETS
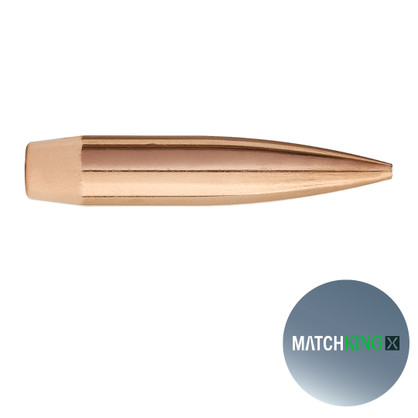
This bullet is perfectly suited for the 25 Creedmoor;
the 131gr Hollow Point Boat Tail design results in tremendous BCs for the shooter.
Age Confirmation

Thank you for your interest in SierraBullets.com.
Our site requires you to be 18 years or older to enter.










