6.3.2 The Assumption
Siacci was concerned with “flat fire” trajectories for small arms. Ballisticians have long distinguished between “flat fire” and “level fire.” When the extended bore line in Figure 6-2 is horizontal or nearly so, we have level fire. For flat fire the initial elevation angle X can have any value. Flat fire means that the downrange elevation angle X is not very much different from X at any point in the useful trajectory of the bullet. In other words, the trajectory does not curve away from the extended bore line very much. This is really a restriction of the maximum range of the gun, and not on the elevation angle of the gun.. The flat fire idea is not valid for mortars or artillery, but it is very useful for rifles and handguns.
Siacci’s assumption for flat fire trajectories was that, since
then
and (6.3-12)
This author has checked this assumption by mathematical computations, and it is very, very good. For modern high powered rifles and bullets with ballistic coefficients of 0.4 or higher, u and v differ by no more than a few tenths of a fps at ranges exceeding 500 yards. So, u can replace v in the drag model with only a very, very small error.
If we substitute equation (6.3-12) into equations (6.3-5) through (6.3-8), the results are:
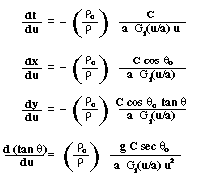(6.3-13) (6.3-14) (6.3-15) (6.3-16)
Notice that q is maintained as a variable. The approximation q » q o is used only in the drag model, where it introduces a negligible error. However, q must be considered a variable in order to maintain accuracy in the calculation of the vertical position y and vertical velocity v y .
Notice also that the differential equations are linear now. Furthermore, equations (6.3-13) and (6.3-14) for time of flight and range are now decoupled . Equations (6.3-15) and (6.3-16) are still coupled and must be solved simultaneously. Initial conditions are given by equations (6.3-9) and the velocity components are given by (6.3-10).